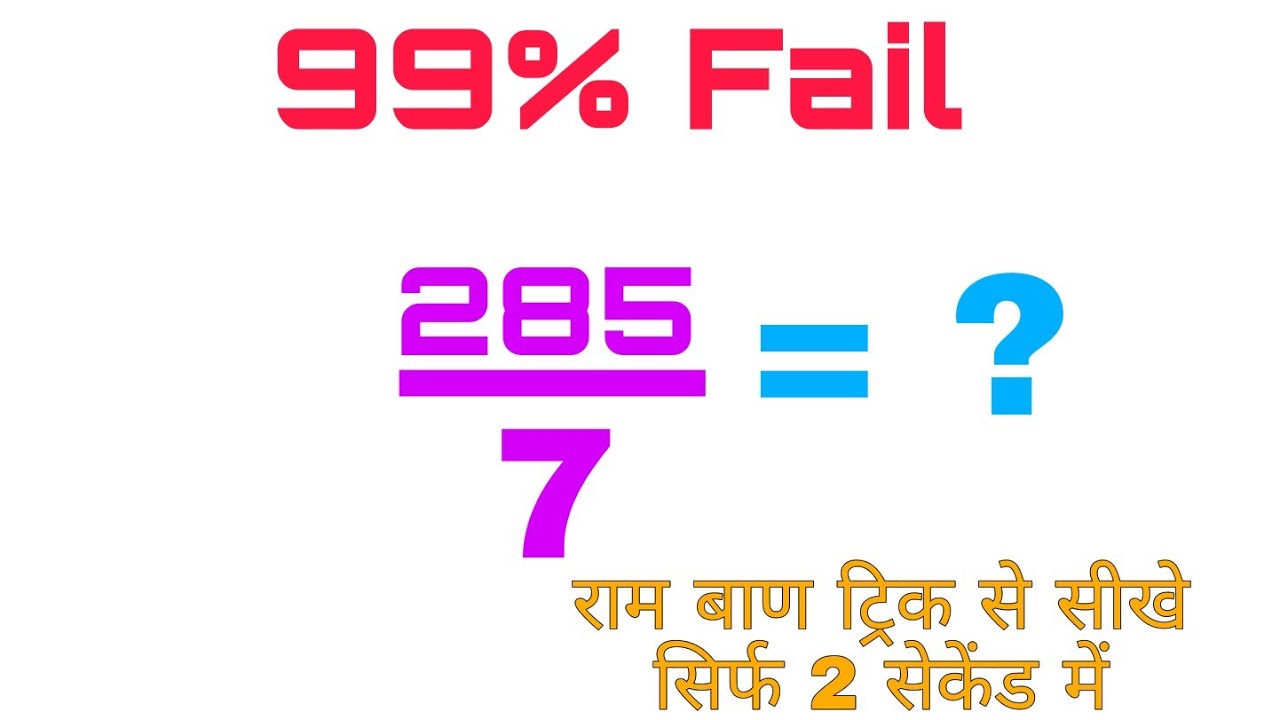
Calculating 285 out of 300: A Simple Division Problem with Wide Application.
The calculation of 285 relative to 300 represents a fundamental arithmetic operation: division. This involves determining how many times 300 goes into 285. Mathematically, it results in a decimal value. For instance, 285 divided by 300 equals 0.95. This decimal can be interpreted as a proportion or a percentage, representing 95% of 300.
The significance of this calculation extends beyond basic arithmetic. It's a cornerstone of various fields. In statistics, it might represent the proportion of successful trials. In business, it could be a measure of efficiency or sales conversion. Even in everyday situations, understanding fractions and proportions allows for better comparison and analysis. The result, 0.95 or 95%, provides context and allows one to make informed conclusions about the relative magnitude of 285 compared to 300.
The exploration of such a basic mathematical operation is pivotal in understanding more complex concepts in fields ranging from finance to data science. This simple division demonstrates the power of fundamental mathematical principles in problem-solving and decision-making across a spectrum of disciplines.
285 divided by 300
Understanding the numerical relationship between 285 and 300 involves examining the quotient obtained from dividing 285 by 300. This calculation yields a decimal value, which, in turn, represents a proportion or percentage. Key aspects of this mathematical operation illuminate crucial implications across various domains.
- Ratio
- Proportion
- Percentage
- Decimal value
- Comparison
- Fraction
- Efficiency
- Success rate
The result, 0.95 (or 95%), represents the proportion of 285 relative to 300. This ratio highlights the comparative relationship between the two numbers. The decimal value reflects this same relationship, demonstrating a connection between different numerical representations. A success rate of 95% implies that 285 out of 300 attempts were successful. The fraction 285/300 mirrors this proportion. The comparison emphasizes the quantitative aspect of the given ratio. Efficiency measures often use this concept to gauge performance. By examining the proportion, one can determine the extent to which one value surpasses another.
1. Ratio
The calculation "285 divided by 300" directly yields a ratio. A ratio expresses the relationship between two quantities. In this instance, the ratio compares the value 285 to the value 300. The result, a decimal value of 0.95, signifies the proportion of 285 relative to 300. This ratio can be expressed as 285:300, a representation of relative size or magnitude. Understanding the ratio of 285 to 300 is critical to making comparative assessments. For instance, the ratio might depict the proportion of successful attempts in a study or the percentage of completed tasks in a project.
The concept of ratio is fundamental in various fields. In manufacturing, the ratio of raw materials to finished goods reveals production efficiency. In finance, the ratio of debt to equity assesses a company's financial health. Analyzing the ratio of 285 to 300 in the context of sales data, for example, can reveal the performance of a particular product line compared to expected outcomes. Furthermore, comprehending the ratio facilitates the understanding of relative magnitudes, which is critical for data analysis and decision-making across diverse disciplines. The ratio serves as a vital tool for comparing different quantities, allowing comparisons to be made quickly and easily, thereby offering a concise and meaningful way to gauge relationships.
In conclusion, the calculation "285 divided by 300" exemplifies a fundamental application of ratio. It demonstrates the importance of ratio in understanding proportions and relative values. This understanding is crucial in various fields, from business and finance to manufacturing and research, and underpins the ability to make informed comparisons and judgments.
2. Proportion
The calculation "285 divided by 300" directly yields a proportion. Proportion describes the comparative relationship between two or more values. In this case, the proportion indicates the extent to which 285 represents a portion of 300. The resulting value, 0.95, signifies that 285 is 95% of 300. This proportional relationship is fundamental in various fields, allowing for meaningful comparisons and analyses.
Proportion is a crucial component of understanding "285 divided by 300" because it reveals the relative size of 285 in comparison to 300. This understanding is essential across diverse applications. In quality control, a proportion might represent the percentage of defect-free products. In economic modeling, it might represent the proportion of a population possessing a certain characteristic. In scientific experiments, a proportion indicates the likelihood of a specific outcome. For example, if 285 out of 300 patients responded favorably to a new medication, the proportion clarifies the treatment's effectiveness. Understanding this proportion is essential for evaluating the medication's potential impact and for future clinical trials. Likewise, in market research, proportion helps in determining the market share of a product or brand. In all these scenarios, the proportional relationship is vital for making sound judgments, driving decisions, and drawing informed conclusions.
In summary, proportion is integral to interpreting "285 divided by 300". The proportional relationship reveals the relative magnitude of 285 within the context of 300. This understanding is vital for analysis, decision-making, and problem-solving in diverse fields. The proportion allows for a clear understanding of the comparative size of 285 in relation to 300, which is a critical component of many quantitative analyses.
3. Percentage
The calculation "285 divided by 300" inherently relates to percentage. A percentage expresses a proportion as a fraction of 100. Understanding the percentage derived from this division offers insight into the relative magnitude of 285 compared to 300, providing a standardized way to express this relationship.
- Interpretation of the Result
The result of dividing 285 by 300 is 0.95. Converting this decimal to a percentage yields 95%. This signifies that 285 is 95% of 300. This interpretation is crucial for comparing and evaluating various data points, particularly when considering benchmarks or performance metrics.
- Application in Real-World Scenarios
A percentage's application spans numerous domains. In business, a 95% completion rate of a project might indicate high efficiency. In education, it could represent student scores on a test. In manufacturing, it might signify the proportion of acceptable products in a batch. Applying this concept to "285 divided by 300" contextualizes the meaning of the result within specific practical situations. For instance, 95% accuracy in a quality control process suggests a robust and dependable system.
- Comparison and Evaluation
Percentage facilitates comparison. By converting proportions into percentages, different data points become easily comparable. For example, if a different project achieved 80% completion, a direct comparison can be made with the 95% completion rate from "285 divided by 300." This comparison allows for assessing relative performance levels, highlighting strengths and weaknesses.
- Standardization and Communication
Percentages provide a standardized way to represent proportions. Using percentages in communicationrather than raw numberssimplifies understanding. This standardization allows for clear and concise communication of findings across various fields. For instance, conveying a result as "95% successful" is more easily understood than simply stating the numbers "285 out of 300." This clarity is essential for efficient communication and effective decision-making.
In conclusion, percentage provides a critical lens through which to interpret the outcome of "285 divided by 300." Converting a decimal result into a percentage facilitates comparison, clarifies meaning, and allows for efficient communication across various disciplines. The standardized nature of percentages contributes significantly to the practical application and comprehension of data analysis.
4. Decimal value
The decimal value derived from dividing 285 by 300, specifically 0.95, is a crucial component of this calculation. It represents the proportion or fraction of 285 relative to 300. The decimal form directly corresponds to the percentage (95%), facilitating comparison and interpretation across various contexts. The decimal 0.95 is a precise representation of this relationship, crucial for nuanced analysis in fields like finance, statistics, and scientific research.
Understanding the decimal value's role in the calculation "285 divided by 300" is vital for practical application. In quality control, a decimal value of 0.95 might signify a high success rate for a production process. In market research, it could represent the market share of a product. In statistical analysis, it might indicate the proportion of successful trials in an experiment. The decimal clearly demonstrates the relationship between the two quantities and its interpretation depends heavily on the specific context. For instance, a decimal value of 0.95 in a medical study might indicate a high efficacy rate of a treatment, while in a manufacturing setting it could signal a low rate of defects.
The significance of understanding decimal values in such calculations cannot be overstated. It provides a standardized, precise representation of relationships. This precision is fundamental for valid comparisons, drawing reliable conclusions, and making informed decisions. Without the decimal representation, the relationship between 285 and 300 remains less easily quantifiable and the full implication of the numerical relationship is less readily apparent. Accurate interpretation of the decimal value is crucial for effective communication and collaboration in various fields, from business to academia.
5. Comparison
The calculation "285 divided by 300" inherently necessitates comparison. This division establishes a relationship between two values, 285 and 300. The resulting quotient (0.95 or 95%) quantifies the relative magnitude of 285 within the context of 300. Comparison, therefore, is not simply an accompanying element; it's fundamental to the calculation's meaning. This comparative aspect allows for the determination of the proportion or fraction represented by 285 out of 300.
Real-world examples illustrate the practical importance of comparison. In quality control, comparing the number of acceptable products (285) to the total produced (300) reveals the success rate. In financial analysis, the comparison of profits (285) to projected profits (300) assesses performance against expectations. In education, comparing student test scores (285) to the possible maximum (300) determines individual and class performance. The understanding of this comparison, facilitated by the division, enables informed decisions and targeted interventions, whether in improving production efficiency or boosting student scores. Furthermore, this comparative analysis aids in determining whether 285 represents a strong or weak result relative to the benchmark of 300, contributing to a nuanced understanding of the data.
In conclusion, the calculation "285 divided by 300" fundamentally hinges on comparison. The resulting proportion, 0.95 or 95%, directly quantifies the relative relationship between 285 and 300. By facilitating this comparison, the calculation offers insights crucial for informed judgments and decisions across diverse domains. This capability is vital, from ensuring quality control to evaluating financial performance and understanding educational outcomes.
6. Fraction
The calculation "285 divided by 300" directly yields a fraction, specifically 285/300. A fraction represents a portion of a whole, and this particular fraction indicates the proportion of 285 out of 300. The fraction's value, 285/300, is equivalent to the decimal 0.95, and represents the same proportional relationship as the percentage, 95%. Understanding this fraction is crucial for interpreting the relative magnitude of 285 compared to 300.
The concept of fractions is fundamental across numerous disciplines. In manufacturing, a fraction might represent the percentage of flawless products within a batch. In finance, it could reflect the proportion of debt to equity. In education, it could illustrate the portion of students who achieved a certain grade level. In all these instances, the fraction provides a concise and precise representation of a portion of a whole, facilitating comparative analysis and informed decision-making. For example, in a survey, if 285 out of 300 respondents favored a particular product, the fraction 285/300 clearly communicates this significant support level and allows for easy comparison with other product preferences.
In summary, the fraction 285/300, arising from the division of 285 by 300, is an integral part of quantifying proportions. Its value, equivalent to 0.95 or 95%, underscores the importance of fractions in interpreting the relative size of quantities. This comprehension is crucial in various fields, from manufacturing and finance to education and research, supporting decision-making processes and offering a clear representation of the portion-to-whole relationship.
7. Efficiency
The calculation "285 divided by 300" reveals a proportion, which, when considered within a context, can illuminate efficiency. This proportion represents a ratio of output to input, a critical factor in evaluating efficiency. The result, 0.95, signifies the extent to which a target or goal is met relative to an established benchmark.
- Resource Utilization
Efficiency, in its broadest sense, encompasses the effective use of resources. A proportion of 285 out of 300 suggests that 95% of available resources were successfully utilized. In a manufacturing setting, this might indicate a high rate of production with minimal waste. In project management, it signifies that a project, or a part of a project, utilized allocated resources to achieve a high percentage of the desired outcomes. The remaining 5% could represent areas needing improvement or adjustments in resource allocation.
- Task Completion Rate
The relationship directly correlates with task completion. If 285 out of 300 tasks are completed, the efficiency is high. This application is relevant across various sectors. In customer service, a high percentage of resolved issues efficiently suggests a well-managed support system. In educational settings, a high completion rate of assignments demonstrates efficient learning strategies. The 5% of tasks not completed warrants further investigation to pinpoint factors impeding completion.
- Process Optimization
The calculation can highlight potential areas for process optimization. If the intended output was 300 units, and 285 were achieved, the 95% efficiency suggests the process is operating effectively. However, a slight deviation indicates potential room for improvement in the operational processes, particularly if the shortfall is consistently observed. Analysis of the areas accounting for the 5% difference may identify bottlenecks or unnecessary steps in the workflow.
- Benchmarking and Comparison
The result facilitates benchmarking against established standards. A comparison to previous performance metrics or industry averages allows evaluation of progress. For instance, if the historical efficiency rate was 90%, the current rate of 95% demonstrates progress and better resource utilization. Understanding benchmarks allows for the identification of best practices and areas needing improvement, enabling continuous improvement.
The calculation, "285 divided by 300," is not merely an arithmetic exercise but a metric for evaluating the efficiency of various processes. The proportion of 0.95 (95%) offers insights into resource allocation, task completion, process optimization, and benchmarks, ultimately helping identify areas requiring attention to maximize productivity and achieve optimal outcomes.
8. Success Rate
The calculation "285 divided by 300" directly relates to success rate. A success rate quantifies the proportion of successful outcomes within a defined set of attempts. The result of 285/300, equivalent to 0.95 or 95%, indicates a high success rate. This success rate signifies the proportion of successful attempts relative to the total attempts, offering a clear measure of performance.
The practical significance of understanding success rate within this context is evident across various domains. In manufacturing, a 95% success rate might indicate a highly efficient production process, minimizing defects and maximizing output. In clinical trials, a 95% success rate for a new drug treatment would suggest its effectiveness and potential efficacy. In educational settings, a high success rate on standardized tests suggests effective teaching methodologies and student engagement. Conversely, a low success rate might indicate areas requiring improvement, be it adjustments to the production process, revisions to a treatment plan, or modifications to instructional strategies. Understanding this success rate, therefore, facilitates informed decision-making, enabling the implementation of corrective measures to boost performance.
In conclusion, the success rate inherent in "285 divided by 300" highlights the critical importance of accurately quantifying outcomes. A high success rate, as exemplified by the calculation, provides a benchmark for evaluating performance and identifying areas needing attention. Understanding this quantitative measure is fundamental in optimizing processes and enhancing outcomes across diverse fields. The 95% success rate, resulting from the division, serves as a concrete metric for evaluating performance, enabling proactive measures to address any issues or inconsistencies present.
Frequently Asked Questions
This section addresses common inquiries regarding the calculation "285 divided by 300," providing clarification and context for this fundamental mathematical operation.
Question 1: What is the result of 285 divided by 300?
The result of dividing 285 by 300 is 0.95. This decimal value represents the proportional relationship between 285 and 300.
Question 2: How is this calculation expressed as a percentage?
Converting the decimal 0.95 to a percentage yields 95%. This indicates that 285 represents 95% of the value 300.
Question 3: What does this calculation mean in a practical context?
The calculation's interpretation depends on the specific context. It might represent a success rate, a proportion of a quantity, or an efficiency measure. Without context, the result remains a mathematical relationship between two numbers.
Question 4: How is the fraction 285/300 related to this calculation?
The fraction 285/300 is equivalent to the decimal 0.95. It represents the same proportional relationship between 285 and 300 as the decimal or percentage.
Question 5: Why is this calculation significant in various fields?
The calculation's significance lies in its ability to express proportions, which are fundamental to quantitative analysis in fields such as business, finance, statistics, quality control, and many others. Its application depends heavily on the context within which it is applied.
In summary, "285 divided by 300" provides a fundamental mathematical relationship. Understanding this relationship, including its decimal, percentage, and fractional representations, is crucial for interpreting data in various fields. The specific meaning depends on the context of application.
The next section will delve into the application of this calculation within specific domains.
Conclusion
The calculation "285 divided by 300" is a fundamental arithmetic operation with profound implications across various fields. This division yields a proportion, expressed as a decimal (0.95) or a percentage (95%), reflecting the relative size of 285 compared to 300. Key takeaways from this exploration include the importance of ratios, proportions, percentages, and fractions in quantifying relationships. The calculation itself demonstrates how numerical relationships can be translated into meaningful contextual interpretations, such as success rates, efficiency measures, or proportions of various quantities. Furthermore, the exploration underscores the significance of clear communication and accurate representation of quantitative data in diverse applications, from manufacturing and business to education and research.
Ultimately, understanding "285 divided by 300" transcends the simple arithmetic operation. It highlights the importance of quantitative analysis in decision-making. Contextualizing this fundamental calculation within specific scenarios allows for the extraction of valuable insights. Careful consideration of the context surrounding numerical relationships is critical to accurate interpretation and effective application of mathematical principles in problem-solving across a broad range of disciplines. The relationship between 285 and 300 serves as a microcosm of the broader process of evaluating and interpreting numerical data to achieve meaningful conclusions.
You Might Also Like
Peyto Exploration Stock: Latest News & AnalysisUnderstanding VUL Vs IUL: Key Differences & Which Is Right For You?
David Ruttenberg: Expert Insights & Strategies
Marc Wolpow: Insights & Strategies For Success
US Quarter Value: How Much Is A Quarter Worth?
Article Recommendations
- Exploring Mydesi Your Ultimate Guide To Indian Culture And Lifestyle
- Moneysideoflifecom Comparisons Evaluating Financial Strategies For A Better Future
- Shiloh Jolie Pitt Boyfriend Personal Life And Relationships